Subscribe our email newsletter for future updates
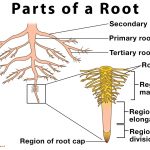 Parts of a Root and Their Functions
Parts of a Root and Their Functions
The root is commonly the underground part of the plant body that helps to anchor it down to the ground and absorbs water
 Types of Deserts With Characteristics and Examples
Types of Deserts With Characteristics and Examples
A desert is a dry, barren landscape which receives an annual rainfall of less than 25 centimeters. Deserts cover about o
 Different Types of Leaves in Plants and Trees
Different Types of Leaves in Plants and Trees
Broadly all leaves are classified into two main types, based on the arrangement of the leaf lamina (the broad, thin, fl
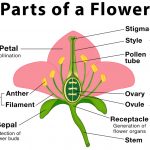 Parts of a Flower With Their Structure and Functions
Parts of a Flower With Their Structure and Functions
Flowers are the reproductive part of a flowering plant. They are the most colorful and attractive organ of a plant body.