Hall Effect

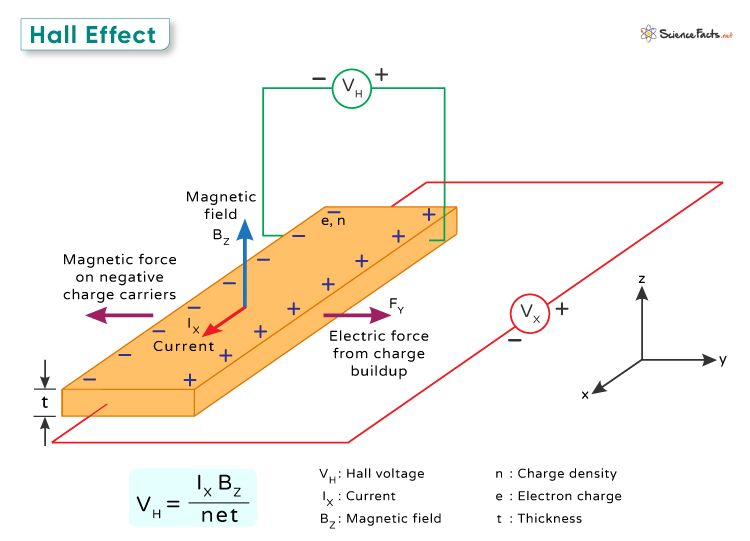
When an electric current flows through a conductor, electrons move along its length in the opposite direction of the current. When a magnetic field is applied perpendicular to this current, something interesting happens. Each electron feels a force called the Lorentz force, which pushes it sideways, perpendicular to both the current direction and the magnetic field lines.

This sideways push is key to understanding the Hall Effect. Imagine electrons moving through a conductor because of an electric field that drives them forward. When a magnetic field is added, it exerts a sideways force on the electrons, making them move to one side of the conductor. As electrons gather on one side, they create a negative charge buildup, leaving a positive charge on the other side. This separation of charges creates an electric field inside the conductor that opposes further electron movement.

This process continues until the electric force from the charge buildup balances the magnetic force from the Lorentz force. The result is a stable situation where the forces are balanced, and a voltage, called the Hall voltage, is generated across the conductor.

In the previous section, we saw how Hall voltage is generated. The following expression gives its value:

Where

• V_H is the Hall voltage
• I_x is the current flowing through the conductor
• B_z is the applied magnetic field
• n is the number of charge carriers per unit volume
• e is the value of the electric charge
• t is the thickness of the conductor

The Hall coefficient is a fundamental parameter in understanding the behavior of charge carriers in materials subjected to a magnetic field. It is defined as the ratio of the Hall voltage to the product of the applied magnetic field, current density, and sample thickness. Mathematically, the Hall coefficient is given by

Where

• R_H is the Hall coefficient
• E_y is the electric field due to the Hall effect
• j_x is the current density

The Hall coefficient provides crucial insights into the material’s electronic properties. Its interpretation extends beyond a mere numerical value. It reflects the density and mobility of charge carriers within the material.

1. Magnetic Field Sensing Equipment: Used to measure magnetic fields accurately in various applications, including scientific research and industrial processes.
2. Current Sensors: Enables the measurement of electrical currents without direct contact, enhancing safety and reliability in electrical systems.
3. Voltage Sensors: Utilized to measure voltages accurately across different systems, ensuring precise monitoring and control.
4. Magneto-Resistive Sensors: Detects changes in magnetic fields by measuring resistance, commonly used in data storage devices, automotive applications, and more.

Aside, Hall effect can determine the carrier concentration, mobility, and conductivity of semiconductors.

Problem 1: A thin rectangular metal plate of thickness 1 cm is subjected to a magnetic field of strength B = 0.5 T. When a current of I = 2 A flows through the plate, a Hall voltage of V_H = 0.1 V is measured. Calculate the charge carrier density in the metal.

Solution

The Hall voltage can be calculated using the formula:

Rearranging the formula for n, we get:

Given data:

• I_x = 2 A
• B_z = 0.5 T
• V_H = 0.1 V
• t = 1 cm = 0.01 m (converted to meters)

Substituting the values, we get:

The charge carrier density of the metal is 6.25 x 10²¹ m^–3.