Brayton Cycle

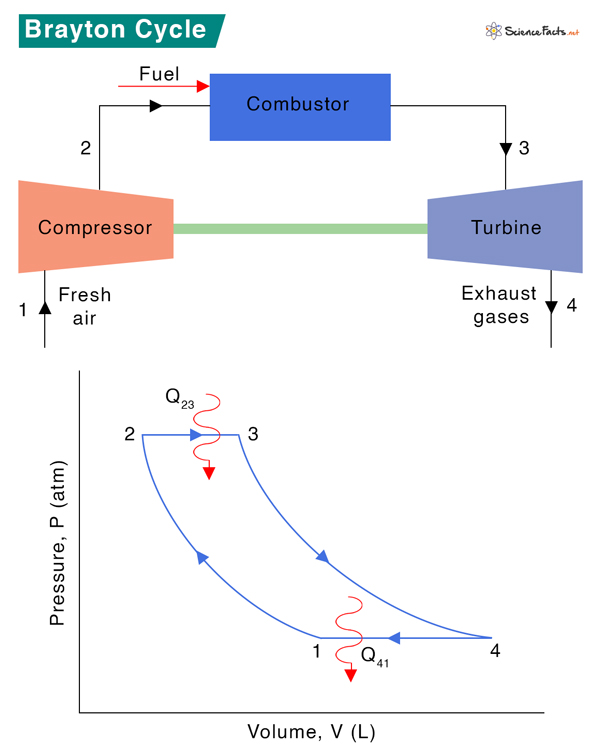
A gas turbine engine consists of three main components: compressor, combustor, and turbine. Air is compressed in the turbine compressor. It is mixed with fuel and combusted under constant pressure in the combustor. The resulting hot gas is made to expand through the turbine to perform work. Most of the work produced in the turbine is utilized to run the compressor. The rest is available to run secondary equipment and generate power. The turbine provides adequate power to drive the compressor and generate mechanical power.

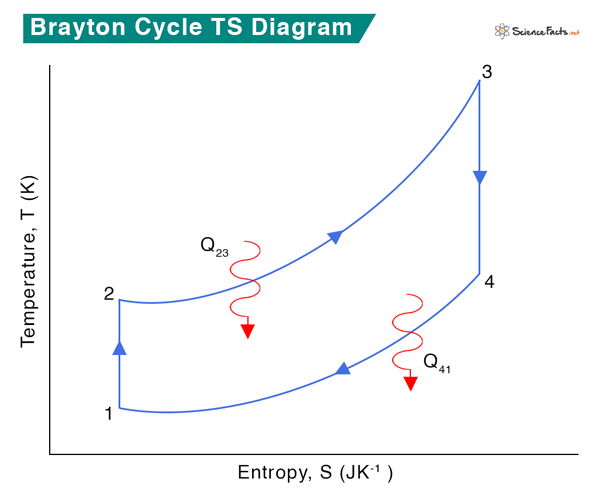
Although the Brayton cycle usually runs as an open cycle system, it is convenient for thermodynamic analysis to assume that the exhaust gases are reutilized in the intake. Therefore, the analysis can be done for a closed system called the ideal Brayton cycle. The ideal Brayton cycle consists of four processes described below with the corresponding PV and TS diagrams.

Process 1 → 2: Isentropic Compression

Fresh ambient air is drawn into the compressor and compressed from pressure P₁ to P₂. The volume reduces from V₁ and V_2, and the temperature increases from T₁ to T₂. The entropy, S, remains constant: S₁ = S₂.

Process 2 → 3: Heat Addition

Fuel and compressed air are sent to the combustor, where fuel burns at constant pressure, P₂ = P₃. Heat is added to the compressor. The temperature rises from T₂ to T₃, volume expands from V₂ to V₃, and entropy increases from S₂ to S₃.

Process 3 → 4 Isentropic Expansion

The heated and compressed gas expands adiabatically through a turbine. The gas works on the turbine’s blades and loses its internal energy, which is equivalent to work that leaves the system. The pressure decreases from P₃ to P₄, temperature decreases from T₃ to T_4, and volume slightly increases from V₃ to V₄. The process is isentropic, which means S₃ = S₄.

Process 4 → 1 Heat Rejection

Isobaric heat rejection occurs in this process at constant pressure, P₄ = P₁. A slight decrease in volume from V₄ to V₁ occurs due to heat rejection. Since it is a heat rejection process, the gas temperature decreases from T₄ to T_1, and entropy reduces from S₄ to S₁.

The general expression for thermal efficiency is given by

Heat is added to during the constant-pressure process 2 → 3 and is given by

Q₂₃ = C_P (T₃ – T₂)

Heat is rejected during the constant-pressure process 4 → 1 and is given by

Q₄₁ = C_P (T₁ – T₄)

Where C_p is the specific heat at constant pressure.

According to the first law of thermodynamics, the net change in internal energy is zero for a closed circle.

Δu = 0

=> (Q₁₂ – W₁₂) + (Q₂₃ – W₂₃) + (Q₃₄ – W₃₄) + (Q₄₁ – W₄₁) = 0

Q₁₂ = Q₃₄ = 0 (since they are adiabatic processes)

Therefore,

Q₂₃ + Q₄₁ – (W₁₂ + W₂₃ + W₃₄ + W₄₁) = 0

=> Q₂₃ + Q₄₁ – W = 0

=> W = Q₂₃ + Q₄₁

Where W = W₁₂ + W₂₃ + W₃₄ + W₄₁ is the net work done.

Therefore,

Adiabatic gas equations give

Where γ is the adiabatic constant. Since P₂ = P₃ and P₄ = P₁ 

The efficiency equation is reduced to 

Here, T₁ is the initial temperature of the undisturbed gas, T₂ is the initial temperature before the combustion, T₃ is the final temperature of the combustion process, and T₄ is the temperature of the gas after it leaves the turbine. The smaller the T₁/T₂ ratio, the higher the efficiency. Therefore, if more heat is added and less heat is lost during the engine operation, the thermal efficiency of the Brayton cycle will be high.

Rewriting the above equation as

\( \frac{P_2}{P_1} \) is called the pressure ratio during compression and is denoted by r_P. 

Therefore,

The pressure ratio in most modern engines ranges from 11 to 16.