Stefan-Boltzmann Law

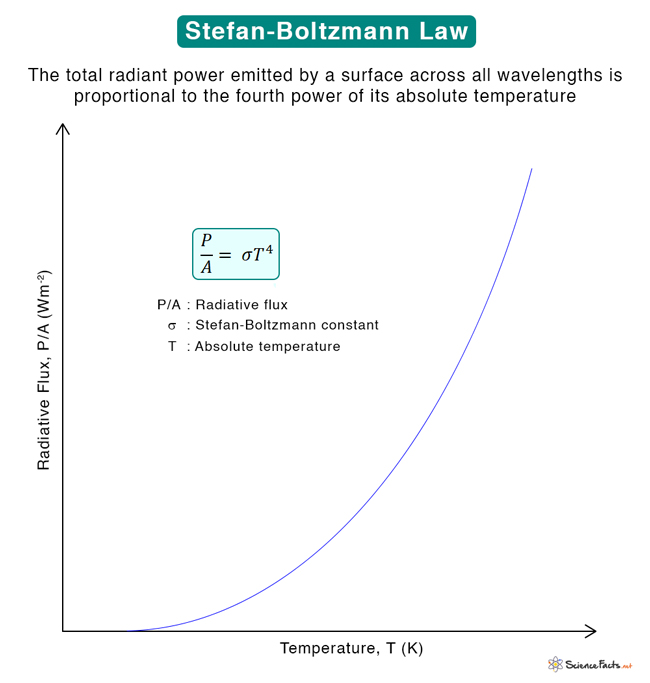
The Stefan-Boltzmann law states that “the total radiant power emitted by a surface across all wavelengths is proportional to the fourth power of its absolute temperature”. The law generally applies to black bodies that absorb all radiation falling on them.

Stefan-Boltzman law is named after Austrian physicists Josef Stefan and Ludwig Boltzmann. Stefan formulated the law in 1877, and Boltzmann derived it in 1884.

Formula

Suppose P is the radiant heat energy emitted per second, A is the surface area of the emitter, and T is the temperature of the surface in absolute scale. According to Stefan-Boltzmann law,

\[ \frac{P}{A} = \sigma T^4 \]

Where s is called the Stefan-Boltzmann constant. It has the following expression:

\[ \sigma = \frac{2p^{5}k_{B}^{4}}{15h^{3}c^{2}} \]

Where

k_B: Boltzmann’s constant

h: Planck’s constant

c: Speed of light

Plugging in the values of k_B, h, and c, the Stefan-Boltzmann constant comes out to be 5.67 x 10^-8 W · m^-2·K^-4.

For objects that are not black bodies, the Stefan-Boltzmann equation modifies to

\[ \frac{P}{A} = e\sigma T^4 \]

Where e is the emissivity of the object. It takes fractional values between 0 and 1. It is 1 for a black body. Therefore, the radiation emitted from an object that is not a black body is less than that emitted by a black body at the same temperature.

Suppose the object radiates energy to its cooler surrounding, which is at a temperature T_C. The radiation equation modifies to

\[ \frac{P}{A} = \sigma (T^4 – T_{C}^4) \]

From the above equations, it is clear that the total radiative power increases as the temperature increases, making heated objects glow bright.

Stefan-Boltzmann’s law can be derived using Planck’s radiation formula. Planck’s equation is integrated over wavelength to get the radiative power.

Application

The Stefan-Boltzmann law can be applied to determine how much power the Sun gives off at its given temperature and how much radiation the Earth receives per second per square meter of its surface.